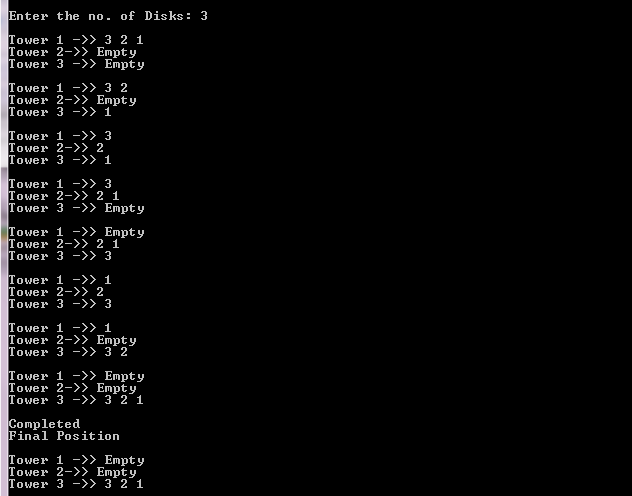
Write a program that displays graphical representation of tower of hanoi. Download Sourcecode for Program that displays graphical representation of tower of hanoi (Size: 2.24 KB) Code for Program that displays graphical representation of tower of hanoi in C++ Programming. Any one have idea how to implement tower of hanoi problem in c. I know the logic but i want to know hot graphically develop it in c. I am trying to implement a Hanoi Tower puzzle with vectors in C++. I specifically have to use vectors, and I am trying to avoid making this into a class. I am pretty sure I have implement most of this correctly, but my tower switches numbers funnily in a couple places.
Easy Tutor author of Program that displays graphical representation of tower of hanoi is from United States. Easy Tutor says Hello Friends,
I am Free Lance Tutor, who helped student in completing their homework.
I have 4 Years of hands on experience on helping student in completing their homework. I also guide them in doing their final year projects.
I have share many programs on this website for everyone to use freely, if you need further assistance, than please contact me on easytutor.2ya [at the rate] gmail [dot] com
I have special discount scheme for providing tutor services. I am providing tutor service to students from various contries, currently most of my students are from United States, India, Australia, Pakistan, Germany, UK and Canada.
I am also here to expand my technical network to receive more opportunity in my career, make friends to help them in resolving their technical problem, learn and share my knowledge, If you like to be my friend, Please send me friend request.
Thanks,
Happy Programming :)
The example Recursively draw a binary tree in C# uses recursion to draw the branches of a tree. Good examples of recursion are hard to come by because the human brain normally thinks iteratively. For example, if you need to paint a fence, you probably think about starting at one end and working your way to the other. You probably don’t think it terms of dividing the fence into two pieces and recursively painting each.
The Tower of Hanoi problem has a good, naturally recursive solution.
Consider the three orange pegs shown in the picture. The goal is to move the pile of green disks from the left peg to another (say the middle peg). You can only move one disk at a time and you can never place a big disk on a smaller disk.
Figuring out how to do this directly is tricky but there is a simple recursive solution. First recursively move every disk except the bottom one to the unused peg (in this case, the right peg). Now there is only one disk on the left peg and no disks on the middle peg so you can move the remaining disk there. Now recursively move the other disks from the right peg to the middle peg.
Tower Of Hanoi Using Recursion
If that’s too confusing, consider a smaller problem with only two disks. To move them from the left to middle peg, first move the top disk to the right peg. Then move the bottom disk to the middle peg. Finally move the top disk to the middle peg. Run the program a few times to see how it works for the larger problem. You can adjust the Delay constant in the code to make the program run slower or faster.
The program stores information about the disks in Stacks. A Stack provides a Push method to add something and a Pop method for removing the most recently added item. The program uses Stacks so it can only add and remove disks from the tops of the pegs. (No sneaking one out of the middle!) The OccupiedPegNum variable keeps track of the Stack that holds all of the disks (when they are not moving).
The following MoveDisks method is the key recursive routine.
MoveDisks takes parameters that tell it where to move the disks from and to, and which peg it can use as “temporary” storage. It also takes a parameter that tells it how many disks to move. To move the disks, the method recursively calls itself to move all but the bottom disk to the “temporary storage” peg, then moves the bottom disk, and then moves the other back from “temporary storage.”
The rest of the program deal with moving the disks from one peg to another and pausing between the moves. It’s not related to the recursive method, which is the main idea in this post, so I’m not going to describe it. Download the example and experiment with it to learn about additional details.